PZE Energy Harvester

 

As wireless sensor nodes become smaller, their energy supply is a limiting factor for further miniaturization as integration density is limited by the space requirements of the energy storage system. MEMS based energy harvesters, such as the one shown in this example, are becoming a key enabler for further miniaturization and deployment of energy autonomous sensor nodes.

Vibration harvesting taps into energy present in moving structures. Several physical effects can be employed to transform mechanical to electrical energy. Piezoelectric, electrostatic or electrodynamic effects are the most common approaches. In this example we show a piezoelectric vibrational energy harvester, similar to the devices shown in Figure 1, fabricated from silicon by a combination of wet and dry etching techniques. This device is designed to be integrated into a energy harvesting module together with a power transfer circuit, a storage element and a resistive load.

Figure 1: Various piezoelectric energy harvesters packaged between two glass substrates. Image courtesy of Holst Centre / IMEC The Netherlands.

Modeling:

A finite element model created in CoventorWare's Designer, comprising a cantilever beam, seismic mass, piezoelectric layer and electrode layers is shown in Figure 2. The cantilever and beam is meshed with 27-node hexahedral elements, the piezo layer with 20-node piezoelectric hexahedral elements. Note that bond pads and conductive path are considered to have a negligible effect on the response and thus omitted in this model. Literature values for the mechanical properties of silicon and the piezoelectric properties of the Aluminium Nitride layer are also used.

Figure 2: CoventorWare Finite element model of a piezoelectric energy harvester showing the mass, cantilever beam, anchor point and PZE layers.

Simulation

CoventorWare's Analyzer modules can be used to simulate the harvester. When a load resistor is directly connected to the harvester, the dissipated power varies over time according to the changing output voltage of the harvester. Of particular interest is the value of load resistance where maximum power transfer from the harvester occurs. To determine the variation of power disspated with load resistance, mechanical boundary conditions were imposed at the anchor point and the harmonic response under a 0.25g load acceleration simulated. The device operates under atmospheric pressure and experiences drag forces under motion. This distributed effect was included in the model by incorporation of Rayleigh (material) damping. The related coefficients were adjusted in order to correctly reflect the quality factor of actual devices operating at atmospheric pressure.

Results

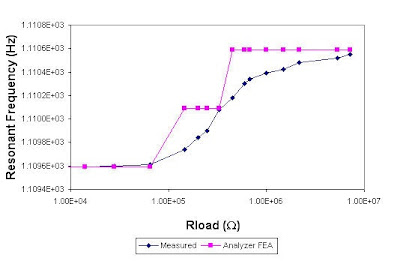
Figure 3 and Figure 4 compare measured and simulated values for average power dissipated and resonant frequency with load resistance, respectively. The measured data accuracy is estimated to be 6%. Predicted power dissipation is within about 25% of the nominal measured values, mesh refinement on selected simulations suggest a reduction to 21% is possible. The remaining difference is likely due to slightly high material damping values used for simulation. Figure 5 shows an animation of harmonic displacement magnitude together with the real voltage on the upper electrode.

Figure 3: Comparison of simulated and measured average power dissipated in a load resistor at 0.25 g acceleration. Note the measured point at Rload of 7.1 Mohm is likely erroneous.

Figure 4: Comparison of simulated and measured shift in resonance frequency at 0.25 g acceleration. The step in the simulated data is due to the 0.25 Hz frequency increment of the harmonic analysis

Figure 5: Animation of harmnonic simulation showing displacement (scaled by a factor of 20) and real voltage developed on the upper piezo surface.

Nombre: Lenny D. Ramirez C.
Asignatura: CRF
Dirección: http://www.coventor.com/mems/applications/PZE_Energy_Harvester.html
Ver blogg: http://lennyramirez-crf3.blogspot.com/